What is Knowledge? Part 2: The Gettier Case

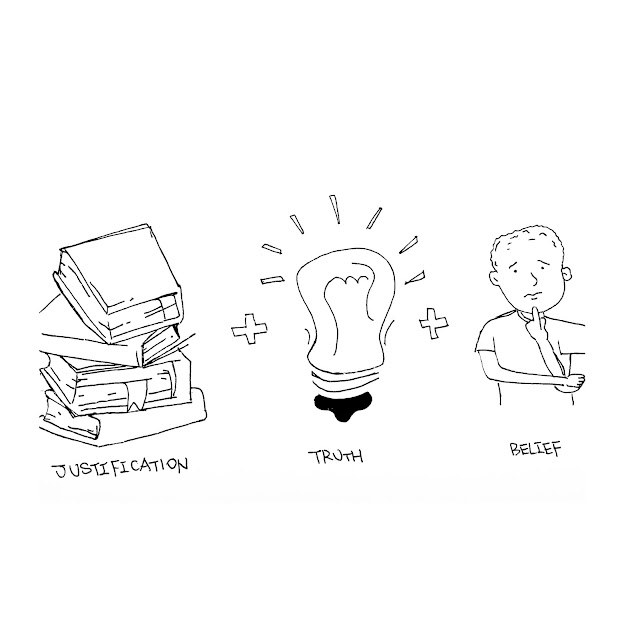
We have previously considered the traditional definition of knowledge as justified true belief.
JTB: S knows that p iff (i) S believes that p; (ii) S's belief that p is justified; and (iii) p is true.
This account of knowledge, however, fails to deal with the famous Gettier problem.

Consider the following story:
Jerry finds a newspaper in his office and starts reading it. He does not notice that the newspaper is yesterday's. On the anthropology section, it says, "The oldest person ever to have lived was Jeanne Calment, a French woman." On this basis, Jerry believes that Jeanne Calment, a French woman, was the oldest person ever to have lived. Jerry then infers, and comes to believe that the oldest person ever to have lived was a woman.
Let p be the proposition that the oldest person ever to have lived was a woman.
Let g be the proposition that the newspaper said that Jeanne Calment, a French woman, was the oldest person ever to have lived.

Suppose just yesterday, anthropologists discovered that a woman, Jenny, is even older than Jeanne. Jenny was the oldest person ever to have lived.

This new detail makes a counterexample to JTB. Let q be the proposition that Jeanne Calment, a French woman, was the oldest person ever to have lived. Jerry believes that q on the basis of grounds g; g justifies his belief that q. Since q logically entails p and Jerry comes to believe that p by deriving p logically from the justified belief that q, Jerry’s belief that p is justified. Since the oldest person ever to have lived, Jenny, was indeed a woman, p is true. According to JTB, Jerry knows that p. Intuitively, however, Jerry does not know that p. It is just a sheer coincidence that Jenny is also a woman so his logically derived belief that p turns out true. Thus, JTB is too permissive; it attributes knowledge where the subject does not know.

We must thus consider a new definition of knowledge.
JTB+NFG:  S knows that p iff (i) S believes that p; (ii) S's belief that p is justified; (iii) p is true; and (iv) S's belief that p is not based on any false grounds.

JTB+NFG successfully rejects this counterexample. Jerry’s belief that p has its justification logically derived from the justified belief that q. Since q is false, Jerry’s belief that p is based on a false ground. This violates condition (iv) of JTB+NFG. According to JTB+NFG, Jerry does not know that p. Thus, JTB+NFG correctly classifies p as not a case of knowledge.